momentum amount of movement

Understand and master the momentum amount of movement:

The amount of movement of an object is defined as the product of its mass by its speed. When two objects collide, the momentum of each varies, but the total momentum of the system remains constant, at least with good approximation.

The variation of the amount of motion of an object is closely related to forces acting thereon. For this reason, one can often determine the average forces involved in a complex phenomenon from changes in amount of movement. forces Related to changes in amount of movement involved in a quantity called the impulse.
IMPULSE AND MOMENTUM
We will define impulse and amount of movement with an example. We then establish a relation between these two variables. the Fig. 7.1 shows a man pushing a cupboard. Let us assume that the frictional forces on the cabinet are negligible. We can establish, using Newton's laws, a relationship between the forces acting on the cabinet,the time during which the forces act and the speed variation of the cabinet.

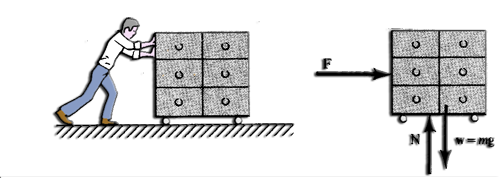
Understand and master the momentum amount of movement

Assume that the force F is exerted on the rack cabinet during a time interval Dt. During this time interval, the speed of the cabinet varies from V0 to V2. By definition, the average acceleration, a, is equal to the variation in speed divided by the time interval: a = (V2- V0)/Dt. Newton's second law relates the average acceleration and the resultant force: F = m.a. We therefore:
or: The impulse (newtons.secondes) is defined as the product of the average Force by the time interval: The momentum (amount of movement) is defined by the product mv, It follows from this definition that the unit of momentum SI is the kilogram x meter per second (kg. M / s). the impulse is equal to the variation of the quantity of motion,, With: P= m.V et P'= m.V'

 

example Its weight is 0.15 kg. If the duration of the impact is 0.001 s, which is the average force exerted on the ball?
The initial momentum of the ball is zero since the ball is stopped.The final amount of movement is P' = m.V'. From the relation F.Dt= P' - P, we deduce that the average force F exerted on the ball is:

 

CONSERVATION OF MOMENTUM:  
Momentum is a very useful concept to study the interaction between two or more objects. Fig. below schematically a collision between two objects. Friction is assumed to be negligible. Each object is subjected to the force of gravity and a normal force.
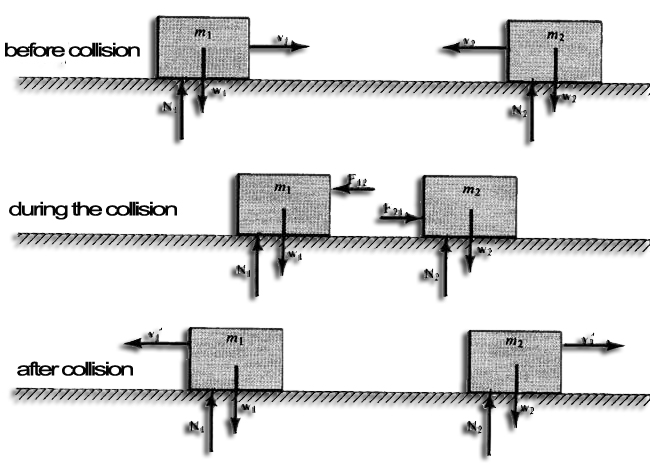Understand and master the momentum amount of movement During the collision, as the objects exert forces on each other. These F 12 and F 21 forces are forces of action and reaction. So they have the same magnitude, but opposite directions. If the collision takes for a time interval Dt, the variation of the amount of movement relative to each object can be calculated from the average forces F12 and F 21 . Since F12 + F21 = 0, if we add the two equations, and This equation shows that the amount of movement resulting from the two objects is unchanged before and after the collision. The total momentum of the system is maintained.. This is important. Indeed, we can relate the speeds of objects before and after the collision without knowing anything about the forces acting between them during it. The fact that these forces are equal, but opposite directions is sufficient to establish the relation.
A cannon mounted on a railroad wagon. This wagon is initially at rest, but it can move without friction . cannon fires a bullet with a mass m = 5 kg and has, relative to the ground, a horizontal speed y = 15 m/ s. The ball moves towards the wall of the wagon.The total weight of the cannon and the wagon is M =15.000 kg. (a) What is the velocity V of the wagon when the ball is in flight? (b) If the ball encrusted in the wall of the wagon, what is the speed of the wagon and the ball after impact?

 

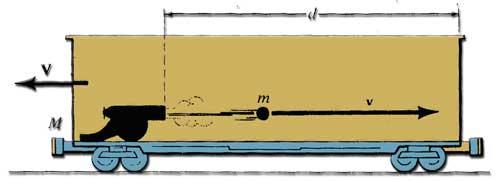
Understand and master the momentum amount of movement

The cannon fires a bullet to the right. The canon is rigidly fixed to the floor of a railway wagon

 

(a) At the fires, the cannon exerts on the ball a force directed to the right. The ball exerts a force equal but opposite on the cannon.The cannon and the wagon are therefore subject a recoil to the left. The total momentum is conserved since there is no external force friction. The momentum before the cannon fires is zero. Therefore, after the fires, the momentum of the ball, which is directed to the right must be equal in magnitude to the momentum of the cannon and of the wagon. laquelle est dirigée vers la gauche. which is directed to the left.
We therefore The recoil velocity of the cannon and of the wagon is very low because their mass is important.
(b) When the ball encrusted in the wall, it exerts a force on it. She is pointing to the right .In turn, the wall exerts a force on the ball, which is directed towards the left. The cannonball and the wagon then stop moving. At this time, the amount of movement, resulting is again zero. Meanwhile, the car was moved to the left and the cannonball to the right.